Below is the online edition of In the Beginning: Compelling Evidence for Creation and the Flood,
by Dr. Walt Brown. Copyright © Center for Scientific Creation. All rights reserved.
Click here to order the hardbound 8th edition (2008) and other materials.
Highly Compressed Solids
A granite cliff on Earth could never be higher than 5 miles. Granite typically has a crushing strength of 2.11 × 108 newtons/meter2 and weighs 26,400 newtons/meter3. Dividing the first number by the second gives 8,000 meters (or 5 miles)—the maximum height before the granite at the base of the cliff is crushed by the load above. (If the entire cliff were under water, buoyancy would allow the cliff to be about 60% higher.)
Let’s examine a more general case and then apply it to several specific examples including the one above. If a tiny cube of any solid is compressed on all six sides by equal pressures (stresses) that exceed the solid’s crushing stress, would the cube be crushed into tiny pieces? No. The confinement pressure is so large and uniform that no tiny fragment of the cube could slip relative to an adjacent fragment. With no shearing stresses in the cube, it would only shrink uniformly, according to Hook’s law: by an amount proportional to the stress. (The subjects of Poisson’s ratio and Mohr’s circle can be skipped with no loss of generality.) Therefore, a fragile glass goblet, or any solid, would not break or be penetrated by water if it were gently placed at the deepest point on an ocean floor, where compressive stresses are gigantic but uniform. Indeed, many of us have seen pictures of delicate, unbroken china in the deep ocean wreckage of the Titanic.
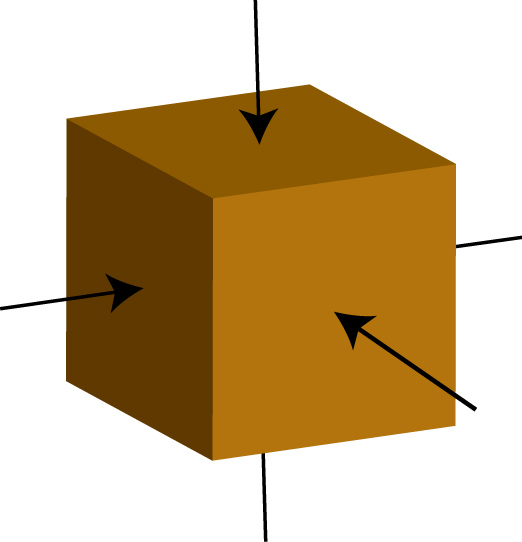
Figure 266: Hydrostatic Compression. Hydrostatic compression occurs when a tiny cube of material (solid or liquid) is compressed equally and uniformly on all six sides, and no stresses lie in the faces of the six sides. Because liquids can sustain no shear stresses, this is automatically true for pressurized liquids. Thus the name “hydro-static” compression. Nevertheless, the term is also used for solids, especially rocks deep in the earth.
The imaginary cube usually has boundaries that are visualized as lying within a larger mass of the same material. Under hydrostatic conditions, no shear stresses exist within the cube. However, with rocks, the compressive forces on the sides of the cube are often unequal, so shear stresses will develop within the cube to try to balance the differences. Those stresses deform the cube to some degree.
For deep rocks, compressive deformations are primarily puttylike, not elastic (or springlike).The greater the compressive deformations, the more the rock deforms and shear stresses disappear—as in a liquid.When drilling into the earth, hydrostatic rocks are encountered at depths of about 5 miles. As drills approach those depths, dense liquids must be added to the drill hole to keep it from collapsing.
Why is a solid not crushed if the compressive stresses exceed the crushing strength and are equal in all directions? The crushing strength of a solid is defined as the axial compression required to fracture a test cylinder of that material. Because there is no external compression on the sides of the test cylinder, internal shearing stresses must develop to try to counter that axial compression. Those shearing stresses, comparable to the axial stresses in magnitude, cause mineral grains to slip at grain boundaries, the weakest part of solids.
If each side of a tiny, confined cube is compressed by large, unequal stresses, internal shearing stresses will develop and deform the original cube. Within the cube are countless grain boundaries between adjacent crystals. If the stresses on the sides of the cube are large and sufficiently different, internal shearing stresses at grain boundaries will break the weakest atomic bonds, produce slippage (dislocations) at grain boundaries, and make the internal stresses nearly hydrostatic. This is putty-like—or plastic—deformation by compression.
The side of a tiny granite cube that was part of a cliff face (or the inside wall of a deep drill hole) would have no compressive stress acting on it. Therefore, internal shearing stresses would try to compensate. If at least 5 miles of granite were loaded above the cube, those shearing stresses would fracture the granite cube, and the pieces would spill out of the cliff face, or into the drill hole.
Other variables affect the compressive strength of solids and the point where plastic deformation by compression begins. The higher the temperature, the weaker the compressive strength. Also, the mere process of deforming a solid generates internal friction that heats and weakens the solid. Defects within crystals are sources of weakness and may produce an early onset of puttylike deformations. Even so, as a general rule, granite 5 miles or more below the Earth’s surface will tend to deform until the compressive loads are almost hydrostatic—equal in all directions and without shear stresses. Any liquid below that solid seal (such as the preflood subterranean ocean) will be trapped, unless forces rupture the seal. High-pressure fluids are often sealed in their containers by gaskets or sealants made of highly-compressed, but malleable, solids.
Most advocates of plate tectonics think of the mantle as a highly viscous liquid. (Textbooks and young minds are filled with this error as well as the belief that the mantle circulates like a liquid.) No, the mantle is almost entirely a solid—a highly compressed solid. [See Figure 96 and “Reasonable Driving Mechanisms” on page 173.]